Printable Poker Hands
DaveChild, 17:23 5 Apr 13. If you mean Ace Ten (suited) then I rated the two (suited and unsuited) fairly evenly and both as 'good' hands. The positional play section was based on several other resources, and I they had Ace Ten in various combinations as a great hand, good hand and even OK hand. Poker Hand Rankings With Printable Cheat Sheet Knowing what beats what in poker or Texas Hold’em is an important early step in learning the game. To help you out, I have provided for you an attractive printable or downloadable “cheat sheet” for both 5 card hand rankings as well as top 24 pre-flop starting hands.
This post works with 5-card Poker hands drawn from a standard deck of 52 cards. The discussion is mostly mathematical, using the Poker hands to illustrate counting techniques and calculation of probabilities
Working with poker hands is an excellent way to illustrate the counting techniques covered previously in this blog – multiplication principle, permutation and combination (also covered here). There are 2,598,960 many possible 5-card Poker hands. Thus the probability of obtaining any one specific hand is 1 in 2,598,960 (roughly 1 in 2.6 million). The probability of obtaining a given type of hands (e.g. three of a kind) is the number of possible hands for that type over 2,598,960. Thus this is primarily a counting exercise.
___________________________________________________________________________
Preliminary Calculation
Usually the order in which the cards are dealt is not important (except in the case of stud poker). Thus the following three examples point to the same poker hand. The only difference is the order in which the cards are dealt.
These are the same hand. Order is not important.
The number of possible 5-card poker hands would then be the same as the number of 5-element subsets of 52 objects. The following is the total number of 5-card poker hands drawn from a standard deck of 52 cards.
The notation is called the binomial coefficient and is pronounced “n choose r”, which is identical to the number of -element subsets of a set with objects. Other notations for are , and . Many calculators have a function for . Of course the calculation can also be done by definition by first calculating factorials.
Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of “3 diamond, 2 heart” hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
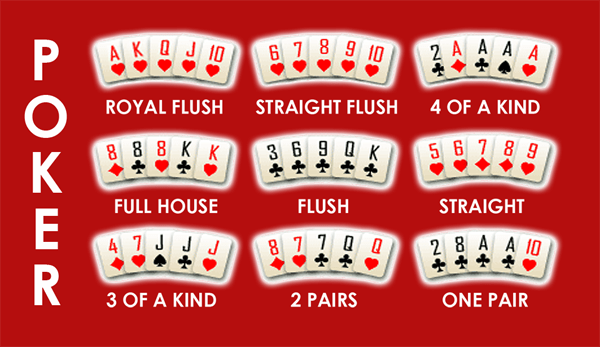
Here’s a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.
The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.
Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let’s fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2’s and choosing 2 cards out of the four 8’s. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.
High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Probabilities of Poker Hands
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
___________________________________________________________________________
2017 – Dan Ma
Only starting out with poker in 2020?
I remember when I started with poker, I found remembering the important parts of the game challenging.
But your journey can become easier with this printable poker cheat sheet for beginners (I wish I had this when starting out!).
Table Of Contents
- How To Use This Texas Holdem Poker Cheat Sheet.
- How To Use This Pot Odds Cheat Sheet – Facing River Bet Example
- How To Use This Pot Odds Cheat Sheet – Facing Flop Bet Example
- Poker Hands Cheat Sheet: Best Texas Hold em Hands
Poker Cheat Sheet For Texas Holdem:
Download the high-quality Poker Cheat Sheet printable (PDF) version:
The cheat sheet includes hyperlinks for further reading on any material you may not yet know.
Click here for more information on pre-flop and post-flop. We also discuss Texas Holdem bet sizing in the highlighted link.
If you like the cheat sheet, you may also enjoy these these awesome starting hand charts from upswing poker. They are a more detailed version of the starting hands section in the cheat sheet above which supplement it nicely. Amazingly they have been downloaded almost 200,000 times!
How To Use This Texas Holdem Poker Cheat Sheet.
Step 1: Find your hand on the chart (example KT suited)
Step 2: Determine whether you should follow coloured or number schematic.
Either:
- If first to raise (no other player has raised before you), follow the coloured schematic.
- If facing a raise or reraise, follow the numbered schematic.
Note: If playing on a 6max table (6 players as opposed to 9), the yellow coloured hands will also be able to be played from any position.
See the image below for the numbered and colour schematic.
Step 3: Take into account information give under headings preflop and post flop.
How to play poker preflop is a tough subject to cover in detail. There are many factors you need to take into account such as:
- Your position and your opponents position.
- Your opponents likely holdings
- Board texture
- Previous history
A brief explanation of why position is powerful and why we play fewer hands when there are more players left to act (still with a hand):
When playing on a fullring table, you will have to contend with nine players, who each have a chance of picking up a big hand. Therefore, when playing a full ring game, you will play fewer hands. You can read more on this concept at fullring vs. 6max.
The difference in player numbers is also why we play a wide range of hands from the Button, but very few hands from UTG (first position). When opening the Button, we only have two players left to act (unlikely for them to have a strong hand), whereas when playing from UTG in a full ring game, eight other players could potentially pick up a big hand.
For more in-depth details on this see Texas Holdem Strategy and Position is King!
Step 4: Take home some cash
Hopefully, this poker cheat sheet will help you ‘bring home the bacon' as they say, but there is always something more to learn in poker. Keep reading for some more cheat sheets which might be of use to you.
Get Your Miniature (Credit Card Sized) Texas Holdem Starting Hands Cheat Sheet
This cheat sheet only contains the most vital information you need so it can handily fit in your pocket. The legends have also been squeezed onto the hand chart in front of hands we always fold.
To download printable PDF which is scaled to credit card size, use the Facebook unlock button:
Poker Odds Cheat Sheet (for Texas Hold'em)
Get your pot odds cheat sheet below. You can use this to determine the number of outs required to continue based on the pot odds you are being offered. You can also use it to convert between percentages, required outs and ratios for all kinds of situations in poker. The pot odds cheat sheet is explained in more detail below:
Click here to get a high-quality printable pdf version of the Poker Odds Cheat Sheet.
When your opponent bets you will be offered odds based on the size of his bet. For example, if your opponent bets half pot you will be offered odds of 3:1 on a call (call 1 to win 3). Essentially, it is your risk to reward ratio.
Pot odds will tell you whether is it correct for you to call or fold based on what size our opponent bet and how many cards that will improve our hand.
If you are interested in the learning poker math, check out our best poker books recommendation page here for some awesome books on poker math.
How To Use This Pot Odds Cheat Sheet – Facing River Bet Example
1. Work out pot odds
In this hand, our opponent bets $26 into a $41.5 pot making the total pot size $67.5. This gives us odds of 67.5: 26 (67.5 = 41.5+26). Or approximately 2.6:1. You can also see how to convert this into a percentage in our article pot odds.
2. Find 2.6:1 on the card (or as close to it as possible).
We locate 2.6:1 on the chart tells us that 2.6:1 translates to 30.11% pot equity. In other words:
- if we win 30% of the time, we will break even,
- if we win > 30% of the time we will make a profit on average in this situation
- if we win <30% of the time, we will make a loss on average in this situation
3. Determine our actual equity
This is the tough part, unfortunately.
You have to estimate how often you are beaten by your opponent in order to determine if you can profitably call or not. To do this you can use a program such as equilab to plug in hands that you think your opponent may have and the hand that you currently hold. To learn more about estimating what your opponent may be holding see the article poker hand range: the comprehensive beginner guide. From the example above, we plug in some hands we think our opponent may have and see that we have 34% equity:
4. Determine if we can profitably call.
Since our equity is greater than our pot odds, we can profitably call the river bet. If our equity were less than the pot odds being offered, we would have to fold as we cannot c call.
How To Use This Pot Odds Cheat Sheet – Facing Flop Bet Example
Let's take a similar situation (confronted with a bet), except this time we are on the flop with KQs, and we have a flush draw with nine outs. A King and Queen which could be considered outs, but they aren't clean outs. This means even if we hit our hand we still may not win (say for example our opponent has AA).
1. Work out equity percentage:
Since we have nine clean outs, we can simply go to the number 9 on the card and then determine our equity.
This means that we need a minimum pot odds of 1.9:1 or 38% when we have nine outs on the flop with two cards still to come.
3. Compare pot odds to odds given by bettor.
Our equity is 38%, so we need pot odds of less than 38%. The lower the pot odds, the more profitable the call.
Our pot odds are 12.5/33 which is 37%, and hence we just about have the pot odds to call. However, we are also in positon (and will act last with more information) and have two overcards to the board (both a King and Queen will make top pair good kicker). So this is an easy call.
4. Further reading
We need seven outs to continue, and we have nine outs with a flush draw. See calculating outs for more details.
Free Printable Poker Hands Chart
For more information on how to use this poker cheat sheet see poker and pot odds.
This video will also be useful to you:
Poker Hands Cheat Sheet: Best Texas Hold em Hands
In case you aren't familiar with the hand strengths, and hand rankings of poker check out the printout Texas Holdem hands cheat sheet:
(You may also be interested in the rules of texas hold em)
There are a few important things to remember when memorising at the poker hand rankings:
Best Five Cards Win
In poker, it is always the best five cards wins. This means it is not only the pairs that matter if there is no clear winner (nobody has a pair), the decision will go down to high card wins.
Kickers
Kickers decide the winning hand when two opponents have the same pair or three of a kind. For example, if one opponent has AQ (ace-queen) and another has AJ, the opponent with AQ would win on an A7522 board as he has the five card hand of AAQ75 whereas the second opponent has AAJ75.
Split Pots
Split pots occur when opponents have the same hand. For example, imagine one opponent has A4 and the other A3 on AQ752 board. Both opponents would have five card hand of AAQ75. Neither the 4 or 3 would play.
Printable Poker Hands Chart
You can get more information about hand rankings on our web page here.
If you are more visually inclined, check out this video on poker hand rankings:
For more on Texas Hold'em strategy, see poker 101.
Make sure you check out the fan favourite posts:
Common poker mistakes & Texas Holdem Poker Tips
Good luck at the poker tables with your new poker cheat sheet!
